Question

A block with mass M attached to a horizontal spring with force constant k is moving with simple harmonic motion having amplitude A1. At the instant when the block passes through its equilibrium position, a lump of putty with mass m is dropped vertically onto the block from a very small height and sticks to it. Part APart complete What should be the value of the putty mass m so that the amplitude after the collision is one-half the original amplitude? Express your answer in terms of the variables M, A1, and k. m = 3M Previous Answers Correct Part B For this value of m, what fraction of the original mechanical energy is converted into heat? Express your answer in terms of the variables M, A1, and k.

Answer 1

Step-by-step explanation:

Given

Mass of block is
`M`

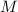
spring constant
`=k`

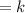
Amplitude is
`A_1`

when putty is placed then amplitude decreases to
`(A_1)/(2)`

Initially
`(1)/(2)kA^2=(1)/(2)Mv^2\quad \ldots(i)`

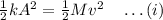
Conserving momentum

`Mv_o=(m+M)v`

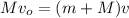
where
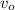`v_o`=initial velocity

`v=(M)/(M+m)v_o`

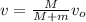
Now

`(1)/(2)k((A_1)/(2))^2=(1)/(2)(M+m)v^2`

`(1)/(2)k((A_1)/(2))^2=(1)/(2)(M+m)((M)/(M+m)v_o)^2\quad \ldots(ii)`

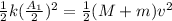
divide (i) and (ii) we get

`(4)/(1)=(M)/(M+m)* ((m+M)/(m))^2`

`4=(m+M)/(M)`

`m=3M`

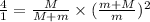
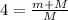
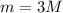
Fraction of energy converted into heat
`=(1)/(2)kA_1^2-(1)/(2)k((A_1)/(2))^2`

`=(1)/(2)kA_1^2[1-(1)/(4)]`

`=(1)/(2)kA_1^2[0.75]`

So,
`(3)/(4)` fraction is converted into heat energy