Answer:
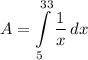

General Formulas and Concepts:
Algebra II
Calculus
Integration
Integration Rule [Fundamental Theorem of Calculus 1]:
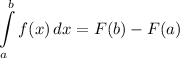
Area of a Region Formula:
![\displaystyle A = \int\limits^b_a {[f(x) - g(x)]} \, dx](https://img.qammunity.org/2021/formulas/mathematics/college/8yomppr4m10wil0api6m0lag5b7hnc5c9y.png)
Explanation:
Step 1: Define
Identify
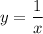
Bounds: [5, 33]
Step 2: Find Area
- Substitute in variables [Area of a Region Formula]:
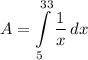
- [Integral] Integrate [Logarithmic Integration]:
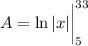
- Evaluate [Integration Rule - Fundamental Theorem of Calculus 1]:
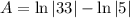
- Condense:

Topic: AP Calculus AB/BC (Calculus I/I + II)
Unit: Integration