Answer:
The risk will be reduced by 0.109
Step-by-step explanation:
Standard deviation for stock A = 49%
Standard deviation for stock B = 49%
Correlation = 0.2
Let's use the standard deviation of portfolio equation:
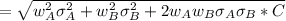
Where
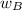 = 100% - 56% = 44%
= 100% - 56% = 44%
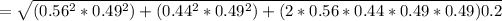
= 0.381 = 38.1%
The risk will be reduced by:
(0.56*0.49)+(0.44*0.49)-0.381
= 0.109