Question

In 1943​, an organization surveyed 1100 adults and​ asked, "Are you a total abstainer​ from, or do you on occasion​ consume, alcoholic​ beverages?" Of the 1100 adults​ surveyed, 429 indicated that they were total abstainers. In a recent​ survey, the same question was asked of 1100 adults and 352 indicated that they were total abstainers. Has the proportion of adults who totally abstain from alcohol​ changed? Use the alphaequals0.10 level of significance.

Answer 1

We conclude that the proportion of adults who totally abstain from alcohol​ has changed.

Explanation:

We are given that in 1943​, an organization surveyed 1100 adults and​ asked, "Are you a total abstainer​ from, or do you on occasion​ consume, alcoholic​ beverages?"

Of the 1100 adults​ surveyed, 429 indicated that they were total abstainers. In a recent​ survey, the same question was asked of 1100 adults and 352 in

Let p = proportion of adults who totally abstain from alcohol.

where, p =
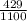`(429)/(1100)` = 0.39

So, Null Hypothesis,
`H_0` : p = 39% {means that the proportion of adults who totally abstain from alcohol​ has not changed}

Alternate Hypothesis,
`H_A` : p
`\\eq` 39% {means that the proportion of adults who totally abstain from alcohol​ has changed}

The test statistics that would be used here One-sample z proportion statistics;

T.S. =
`\frac{\hat p-p}{\sqrt{(\hat p(1-\hat p))/(n) } }` ~ N(0,1)

where,
`\hat p` = sample proportion of adults who totally abstain from alcohol =
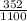`(352)/(1100)` = 0.32

n = sample of adults surveyed = 1100

So, test statistics =
`\frac{0.32-0.39}{\sqrt{(0.32(1-0.32))/(1100) } }`

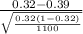
= -4.976

The value of z test statistics is -4.976.

Now, at 0.10 significance level the z table gives critical values of -1.645 and 1.645 for two-tailed test.

Since our test statistics doesn't lie within the range of critical values of z, so we have sufficient evidence to reject our null hypothesis as it will fall in the rejection region due to which we reject our null hypothesis.

Therefore, we conclude that the proportion of adults who totally abstain from alcohol​ has changed.