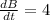Answer:
Explanation:
Derivative indicates rate of change of dependent variable with respect to independent variables. It indicates the slope of a line that is tangent to the curve at the specific point.
Given:
Number of bees is modeled by the function
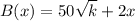
The daily high temperature is increasing at a rate of 2 °F per day when the number of bees spotted is 100.
To find:
rate of change of number of bees when 100 bees are spotted
Solution:
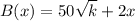
Differentiate with respect to t,

Put
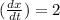

At x = 100,