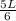Question

A long uniform wooden board (a playground see-saw) has a pivot point at its center. An older child of mass M=32 kg is sitting a distance L from the pivot. On the other side of the pivot point are two smaller children each of mass M/2. One is sitting a distance L/6 from the pivot. How far from the pivot must the other small child be sitting in order for the system to be balanced?

Answer 1

Answer:
`(5L)/(6)`

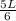
Step-by-step explanation:

Given

Wooden board is pivoted at center and

Older child of mass
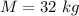`M=32\ kg` is sitting at a distance of L from center

if two child of mass
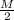`(M)/(2)` is sitting at a distance
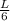`(L)/(6)` and
`x`(say) from pivot then net torque about pivot is zero

i.e.

`\Rightarrow \tau_(net)=MgL-(M)/(2)g(L)/(6)-(M)/(2)gx`

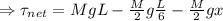
as
`\tau_(net)=0`

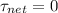
Therefore

`MgL=(M)/(2)g(L)/(6)+(M)/(2)gx`

`L-(L)/(6)=x`

`x=(5L)/(6)`

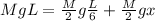
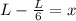
Therefore another child is sitting at a distance of
`(5L)/(6)`