Question

A well-known battery manufacturer claims its product lasts at least 5000 hours, on average. If a sample of 81 batteries has an average lifetime of 4917.5 hours with a standard deviation of 450 hours, use the critical value approach to determine whether you reject or not reject the null hypothesis at a 5% level of significance. What does this mean in terms of the manufacturer's claim

Answer 1

`t=(4917.5-5000)/((450)/(√(81)))=-1.65`

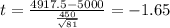
The degrees of freedom for this case are:

`df=n-1=81-1=80`

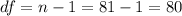
We need to find a critical value in the t distribution with 80 degrees of freedom who accumulates 0.05 of the area in the left and we got:

`t_(cric)= -1.664`

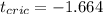
Since the calculated value is not less than the critical value we don't have enough evidence to conlcude that the true mean is significantly lower than 5000 hours. Then the claim by the manufacturer (product lasts at least 5000 hours) makes sense.

Explanation:

Information given

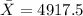`\bar X=4917.5` represent the sample mean

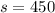`s=450` represent the sample standard deviation

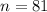`n=81` sample size

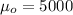`\mu_o =5000` represent the value to check

`\alpha=0.05` represent the significance level

t would represent the statistic (variable of interest)

System of hypothesis

We want to determine if product lasts at least 5000 hours, the system of hypothesis would be:

Null hypothesis:
`\mu \geq 5000`

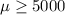
Alternative hypothesis:
`\mu < 5000`

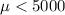
The statistic for a one sample t testo for the true mean is given by:

`t=(\bar X-\mu_o)/((s)/(√(n)))` (1)

Replacing the info given we got:

`t=(4917.5-5000)/((450)/(√(81)))=-1.65`

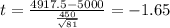
The degrees of freedom for this case are:

`df=n-1=81-1=80`

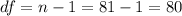
We need to find a critical value in the t distribution with 80 degrees of freedom who accumulates 0.05 of the area in the left and we got:

`t_(cric)= -1.664`

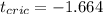
Since the calculated value is not less than the critical value we don't have enough evidence to conlcude that the true mean is significantly lower than 5000 hours. Then the claim by the manufacturer (product lasts at least 5000 hours) makes sense.