Question

Segment KL is a diameter of circle Q. Circle Q is represented by the equation (x − 11)2 + (y + 15)2 = 7. What is KL?

A. 98

B. 14

C. 2 radical 7

D. radical 14

Answer 1

We have been given that segment KL is a diameter of circle Q. Circle Q is represented by the equation
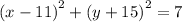`\left(x-11\right)^2+\left(y+15\right)^2=7`. We are asked to find the length of KL.

We know that standard equation of a circle is in form
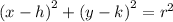`\left(x-h\right)^2+\left(y-k\right)^2=r^2`, where point (h,k) represents center of circle and r represents radius of circle.

Upon comparing our given equation with standard equation of circle, we can see that the center of the circle is at point
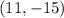`(11,-15)` and
`r^2=7`.

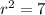Let us solve for r by taking positive square root.

`√(r^2)=√(7)`

`r=√(7)`

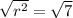
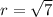
We know that diameter is two times radius, so value of diameter KL would be 2 times r.

`KL=2\cdot √(7)`

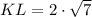
Therefore, the volume of KL is
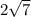`2√(7)` and option C is the correct choice.