Answer:

Explanation:
For the surface area we need to add up all the areas in the pyramid:
- area of the triangle sides (there are 4 triangles)
Area of the base:
the base is a square, and the area of a square is given by:
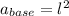
where
 is the length of the side:
is the length of the side:
 , thus:
, thus:
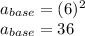
Area of the triangles:
one triangle has the area given by the formula:
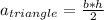
where
 is the base of the triangle:
is the base of the triangle:

and
 is the height of the triangle:
is the height of the triangle:
 , thus we have the following:
, thus we have the following:
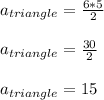
the expression that represents the surface area of the pyramid is:
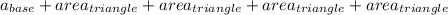
substituting our values:

which is option B