Question

MODELING WITH MATHEMATICS The population P (in thousands) of Austin, Texas, during a recent decade can be approximated by y = 494.29 * (1.03) ^ f , where is the number of years since the beginning of the decade. a. Tell whether the model represents exponential growth or exponential decay b. Identify the annual percent increase or decrease in population . Estimate when the population was about 590,000 .

Show work

Answer 1

a) growth

b) 3%

c) 6 years (since the beginning of the decade)

Explanation:

Given:

The population P (in thousands) of Austin, Texas during a recent decade can be approximated by
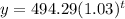`y=494.29(1.03)^t` when t is the number of years since the beginning of the decade.

General form of an exponential function:
`y=ab^x`

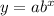
where:

• a is the y-intercept (or initial value)
• b is the base (or growth factor)
• x is the independent variable
• y is the dependent variable

If
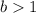`b > 1` then it is an increasing function

If
`0 < b < 1` then it is a decreasing function

a) The model represents exponential growth as 1.03 > 1

b) The annual percent increase of the population is 3%

1.03 - 1 = 0.03

0.03 x 100 = 3%

c) To estimate when was population about 590,000 set y = 590 and solve for t:

`\implies 590=494.29(1.03)^t`

`\implies (590)/(494.29)=(1.03)^t`

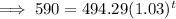
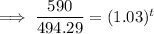
Take natural logs of both sides:

`\implies \ln\left((590)/(494.29)\right)=\ln (1.03)^t`

`\implies \ln\left((590)/(494.29)\right)=t\ln (1.03)`

`\implies t=(\ln\left((590)/(494.29)\right))/(\ln (1.03))`

`\implies t=5.988069001...`

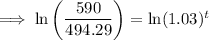
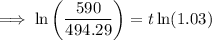
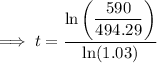
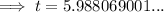
Therefore the population was about 590,000 6 years since the beginning of the decade.