Question

What is the remainder when (x^3+1) is divided by (x^2-x+1)?

Answer 1

A; x + 1

Explanation:

Since the viniculum in a fraction represents division, we can rewrite the expression like this:

`(x^3+1)/(x^2-x+1)`

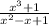
We can already see that
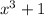`x^(3) + 1` could be factored:

Since anything with a "one" as its base will remain one, we can rewrite:

`x^3+1^3`

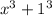
Now, we can apply the "sum of cubes" formula:

`(\left(x+1\right)\left(x^2-x+1\right))/(x^2-x+1)`

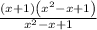
Cancelling out!

`x+1`

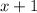
Thusly, you are correct and the remainder is
`\boxed{x+1{\text{\:or\:A}}}`.

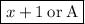Hope this helps! ((: