Answer:
The probability that she will not pull lipstick is 71%.
Explanation:
The probability of an event E is the ratio of the favorable number of outcomes to the total number of outcomes.
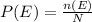
Here,
n (E) = favorable number of outcomes
N = total number of outcomes
The contents in Mrs. Braddock's bag are:
Number of lipsticks = n (L) = 6
Number of eye shadows = n (S) = 4
Number of eye liners = n (E) = 6
Number of mascaras = n (M) = 5
Total number of items in the bag = N = 21
Consider that the probability of an event occurring is P. Then the probability of the given event not taking place is known as the complement of that event.
Complement of the given event is, 1 – P.
Compute the probability of selecting a lipstick as follows:
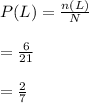
Compute the probability of not selecting a lipstick as follows:
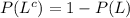
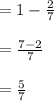
Convert this probability into percentage as follows:
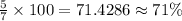
Thus, the probability that she will not pull lipstick is 71%.