Answer:
The radius of hole is 5 feet
Explanation:
Depth of conical hole = 9 feet
Let the radius of hole be r
Volume of conical hole =
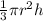
So, Volume of conical hole =
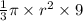
We are given that volume of a CONE-shaped hole is 75pi ft cubed.
So,
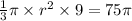
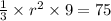
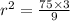
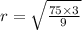
r=5
Hence The radius of hole is 5 feet