Question

Which statements about the ellipse are true? Check all that apply.

The center is located at (2, –1).

The major axis is 8 units long.

The minor axis is 3 units long.

The vertices are 4 units above and below the center.

The foci are units above and below the center.

The foci are located along a horizontal l

Answer 1

1 2 4 5

Explanation:

edg 2021

Answer 2

The center is located at (2, –1).

The major axis is 8 units long.

The vertices are 4 units above and below the centre.

The foci are √7 units above and below the centre.

Explanation:

Assume the ellipse looks like the one below.

The properties of a vertical ellipse are

`\textbf{Vertical ellipse}\\( (x - h)^(2) )/(b^(2)) + ((y - k)^(2))/(a^(2)) = 1\begin{cases}\text{Centre} = (h,k)\\\text{Dist. between vertices} = 2a\\\text{Vertices} = (h, k\pm a)\\\text{Dist. between covertices} = 2b\\ \text{Covertices}= (h\pm b, k)\\c = \sqrt{a^(2) - b^(2)}\\\text{Dist. of foci from centre} = c\\\text{Foci} = (h, k\pm c)\\\end{cases}`

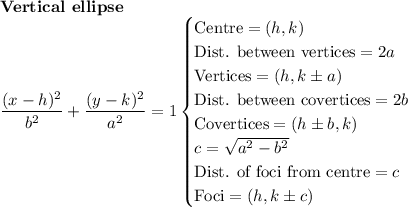
A. Centre

TRUE. The centre is at (2,-1).

B. Major axis

TRUE

Length of major axis = 3 - (-5) = 3 + 5 = 8

C. Minor axis

False

Length of minor axis = 5 - (-1) = 5 + 1 = 6

D. Vertices

TRUE

Distance between vertices = 8 = 2a

a - 8/2 = 4

Vertices at (h, k ± a) = (2, -1 ± 4)

E. Foci

TRUE

c² = a² - b² = 4² - 3² = 16 - 9 = 7

c = √7

The foci are √7 above and below the centre.

F. Foci

False.

The foci are on a vertical line.