Question

Orlando invests $1000 at 6% annual interest compounded daily and Bernadette invests $1000 at 7%

simple interest. After how many whole years will Orlando's investments be worth more than
Bernadette's investments?

Answer 1

6 Years

Explanation:

Orlando invests $1000 at 6% annual interest compounded daily.

Orlando's investment =
`A=1000(1+(0.06)/(365))^((365* t))`

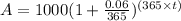
Bernadette invests $1000 at 7% simple interest.

Bernadette's investment = A = 1000(1+0.07×t)

By trail and error method we will use t = 5

Bernadette's investment will be after 5 years

1000(1 + 0.07 × 5)

= 1000(1 + 0.35)

= 1000 × 1.35

= $1350

Orlando's investment after 5 years

`A=1000(1+(0.06)/(365))^((365* 5))`

=
`1000(1+0.000164)^(1825)`

=
`1000(1.000164)^(1825)`

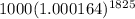
= 1000(1.349826)

= 1349.825527 ≈ $1349.83

After 5 years Orlando's investment will not be more than Bernadette's.

Therefore, when we use t = 6

After 6 years Orlando's investment will be = $1433.29

and Bernadette's investment will be = $1420

So, after 6 whole years Orlando's investment will be worth more than Bernadette's investment.