Question

Suppose Kristen is researching failures in the restaurant business. In the city where she lives, the probability that an independent restaurant will fail in the first year is 32 % . She obtains a random sample of 72 independent restaurants that opened in her city more than one year ago and determines if each one had closed within a year. What are the mean and standard deviation of the number of restaurants that failed within a year? Please give your answers precise to two decimal places.

Answer 1

The mean of the number of restaurants that failed within a year is 23.04 and the standard deviation is 3.96.

Explanation:

For each restaurant, there are only two possible outcomes. Either it fails during the first year, or it does not. The probability of a restaurant failling during the first year is independent of other restaurants. So we use the binomial probability distribution to solve this question.

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

The expected value of the binomial distribution is:

`E(X) = np`

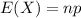
The standard deviation of the binomial distribution is:

`√(V(X)) = √(np(1-p))`

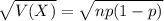
The probability that an independent restaurant will fail in the first year is 32%.

This means that
`p = 0.32`

72 independent restaurants

This means that
`n = 72`

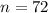
Mean:

`E(X) = np = 72*0.32 = 23.04`

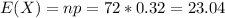
Standard deviation:

`√(V(X)) = √(np(1-p)) = √(72*0.32*0.68) = 3.96`

The mean of the number of restaurants that failed within a year is 23.04 and the standard deviation is 3.96.