Question

Write an expression in simplest form for the perimeter of a right triangle with leg lengths of 3a cubed and 4a cubed

Answer 1

106.4622
`a^(3)`

Explanation:

Given the information:

• A right triangle

• Leg lengths of
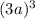  `(3a)^(3)` and
  `(4a)^(3)`

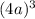
Use the pytagon theory to find the hypotenuse of the triangle

`a^(2) + b^(2) = c^(2)`

<=>
`((3a)^(3)) ^(2) + ((4a)^(3)) ^(2) = c^(2)`

<=>
`(3a)^(6) + (4a)^(6) = c^(2)`

<=>
`c^(2) = 4285a^(6)`

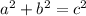
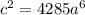
Take the square root of both sides

<=> c = 69.4622
`a^(3)`

=> expression in simplest form for the perimeter of a right triangle is:

`(3a)^(3)` +
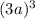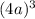`(4a)^(3)` + 69.4622
`a^(3)`

= 27
`a^(3)` + 64
`a^(3)` + 69.4622
`a^(3)`

= 106.4622
`a^(3)`