Answer:
0 is an inflection point
1/4 is a local maximum.
Explanation:
To begin with you find the first derivative of the function and get that
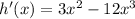
to find the critical points you equal the first derivative to 0 and get that
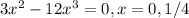
To find if they are maximums or local minimums you use the second derivative.
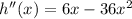
since
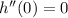 is neither an inflection point, and since
is neither an inflection point, and since
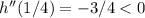 then 1/4 is a maximum.
then 1/4 is a maximum.