Question

(Solidification) You are performing a double slit experiment very similar to the one from DLby shining a laser on two narrow slits spaced 7.5 × 10-5meters apart. However, by placing a piece of crystal in one of the slits, you are able to make it so that the rays of light that travel through the two slits are πout of phasewith each other (that is to say,Δφ!=휋).If you observe that on a screen placed 4meters from the two slits that the distance between the bright spot closest to the center of the interference pattern and the center of the pattern is 1.5 cm, what is the wavelength of the laser? (Just as in DL, you may use the small angle approximation sinθ ≈ θ ≈ tanθ.)

Answer 1

The wavelength of the laser, λ = 5.625 * 10⁻⁷ m

Step-by-step explanation:

Separation of the narrow slits, d = 7.5 * 10⁻⁵ m

The distance between the screen and the two slits, d = 4m

The distance between the bright spot and the center of the pattern, Y = 1.5 cm

Y = 1.5 * 10⁻² m

To calculate the wavelength, λ, of the laser we will use the relationship:

`Y = (\lambda L)/(2d) \\1.5 * 10^(-2) = (\lambda * 4)/(2*7.5*10^(-5) )\\\lambda = (1.5 * 10^(-2) * 15 * 10^(-5) )/(4)`

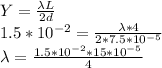
λ = 5.625 * 10⁻⁷ m