Question

.In the Star Wars franchise, Yoda stands at only 66 centimeters tall. Suppose you want to see whether or not hobbits from the Lord Of The Rings are taller than Yoda, on average. From prior research you know that the distribution of hobbit heights are approximately Normally distributed. From a sample of 7 hobbits, you find their mean height ¯ x = 80cm with standard deviation s = 10.8cm. Does sample evidence suggest at the α = 0.01 level of significance that the average hobbit is taller than Yoda? Use steps A through F to test the appropriate hypotheses.

Answer 1

We conclude that the average height of hobbit is taller than Yoda.

Explanation:

We are given that in the Star Wars franchise, Yoda stands at only 66 centimetres tall.

From a sample of 7 hobbits, you find their mean height
`\bar X` = 80 cm with standard deviation s = 10.8 cm.

Let
`\mu` = average height of hobbit.

So, Null Hypothesis,
`H_0` :
`\mu`
`\leq` 66 cm {means that the average height of hobbit is shorter than or equal to Yoda}

Alternate Hypothesis,
`H_A` :
`\mu` > 66 cm {means that the average height of hobbit is taller than Yoda}

The test statistics that would be used here One-sample t test statistics as we don't know about population standard deviation;

T.S. =
`(\bar X-\mu)/((s)/(√(n)))` ~
`t_n_-_1`

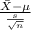where,
`\bar X` = sample mean height = 80 cm

s = sample standard deviation = 10.8 cm

n = sample of hobbits = 7

So, test statistics =
`(80-66)/((10.8)/(√(7)))` ~
`t_6`

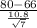= 3.429

The value of t test statistics is 3.429.

Now, at 0.01 significance level the t table gives critical value of 3.143 for right-tailed test. Since our test statistics is more than the critical value of t as 3.429 > 3.143, so we have sufficient evidence to reject our null hypothesis as it will fall in the rejection region due to which we reject our null hypothesis.

Therefore, we conclude that the average height of hobbit is taller than Yoda.