Question

​Claim: Most adults would erase all of their personal information online if they could. A software firm survey of 640 randomly selected adults showed that 99.2​% of them would erase all of their personal information online if they could. Make a subjective estimate to decide whether the results are significantly low or significantly​ high, then state a conclusion about the original claim.

Answer 1

Given:

Sample size, n = 640

Since result showed that 99.2​% of them would erase all of their personal information online if they could, we have:

P' = 99.2% = 0.992

Let's take p = 50%

Our null and alternative hypothesis will be :

H0 : u = 0.5

H1 : u > 0.5

In this case we are to check for the test statistic.

`(P' - p)/(√(p(1-p)/n))`

`= (0.992 - 50)/(√(50(1-50)/640))`

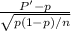
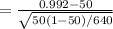
= 24.9

Test statistic, Z = 24.893

In conclusion, the results are significantly high, which suggets there is suffcient evidence to support the claim that most adults would erase all of their personal information online.