Answer:
The probability that
 is, approximately, 0.3594 or about 35.94% (or simply 36%).
is, approximately, 0.3594 or about 35.94% (or simply 36%).
Explanation:
Firstly, we have to know that the random variable, in this case, is normally distributed. A normal distribution is completely determined by its two parameters, namely, the population mean and the population standard deviation. For the case in question, we have a mean of
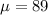 , and a standard deviation of
, and a standard deviation of
 .
.
To find the probability in question, we can use the standard normal distribution, a special case of a normal distribution with mean equals 0 and a standard deviation that equals 1.
All we have to do is "transform" the value of the raw score (x in this case) into its equivalent z-score. In other words, we first standardize the value x, and then we can find the corresponding probability.
With this value, we can consult the cumulative standard normal table, available in most Statistics textbooks or on the Internet. We can also make use of technology and find this probability using statistical packages, spreadsheets, and even calculators.
The corresponding z-score for a raw score is given by the formula:
 [1]
[1]
And it tells us the distance of the raw score from the mean in standard deviations units. A positive value of the z-score indicates that the raw value is above the mean. Conversely, a negative value tells us that the raw score is below the mean.
With all this information, we are prepared to answer the question.
Finding the probability

The corresponding z-score
According to formula [1], the z-score, or standardized value, is as follows:

From the question, we already know that
x = 85
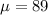

Thus

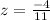
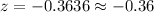
Consult the probability using the cumulative standard normal table
With this value for z = -0.36, we can consult the cumulative standard normal table. The entry for use it is this z-score with two decimals. This z-score tells us that the raw value of 85 is 0.36 standard deviations below from the population mean.
For z = -0.36, we can see, in the table, an initial entry of -0.3 at the first column of it. We then need to find, in the first line (or row) of the table, the corresponding 0.06 decimal value remaining. With this two values, we can determine that the cumulative probability is, approximately:
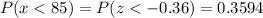
Then,
 or, in words, the probability that
or, in words, the probability that
 is, approximately, 0.3594 or about 35.94% (or simply 36%).
is, approximately, 0.3594 or about 35.94% (or simply 36%).
Remember that this probability is approximated since we have to round the value of z to two decimal places (z = -0.36), and not (z = -0.3636), because of the restrictions to two decimals places for z of the standard normal table. A more precise result is
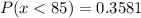 using technology, shown in the graph below.
using technology, shown in the graph below.
Notice that, in the case that the cumulative standard normal table does not present negative values for z, we can use the next property of the normal distributions, mainly because of the symmetry of this family of distributions.
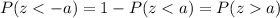
For the case presented here, we have

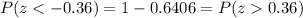
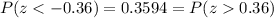
Which is the same probability obtained in the previous step.
The graph below shows the shaded area for the probability of
 finally obtained.
finally obtained.