We have been given that the value of Vishal's car is depreciating exponentially. The relationship between V, the value of his car, in dollars, and t, the elapsed time, in years, since he purchased the car is modeled by the equation
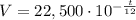 . We are asked to find the time, when Vishal's car will be worth $10,000.
. We are asked to find the time, when Vishal's car will be worth $10,000.
To find the time, we will equate our given equation with 10,000 as:
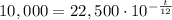

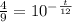
Now we will take log on both sides.
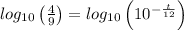
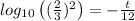


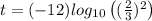
Using property
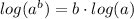 , we will get:
, we will get:
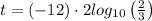

Therefore, the value of Vishal's car will be $10,000 after approximately
 years.
years.