Answer:
99 dB
Step-by-step explanation:
To find the new sound intensity level you calculate first the initial intensity by using the following formula:
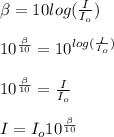
where β is the sound level of 79dB and Io is the hearing threshold of 10^-12 W/m^2. By replacing you obtain:
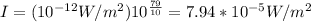
The new sound intensity level is given by:
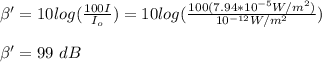
hence, the answer is 99 dB