Question

A sphere of radius 5.00 cm carries charge 3.00 nC. Calculate the electric-field magnitude at a distance 4.00 cm from the center of the sphere and at a distance 6.00 cm from the center of the sphere if the sphere is a solid insulator with the charge spread uniformly throughout its volume. Express your answers in newtons per coulomb separated by a comma. Repeat part A if the sphere is a solid conductor.

Answer 1

a) E = 8.63 10³ N /C, E = 7.49 10³ N/C

b) E= 0 N/C, E = 7.49 10³ N/C

Step-by-step explanation:

a) For this exercise we can use Gauss's law

Ф = ∫ E. dA =
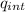`q_(int)` /ε₀

We must take a Gaussian surface in a spherical shape. In this way the line of the electric field and the radi of the sphere are parallel by which the scalar product is reduced to the algebraic product

The area of ​​a sphere is

A = 4π r²

if we use the concept of density

ρ = q_{int} / V

q_{int} = ρ V

the volume of the sphere is

V = 4/3 π r³

we substitute

E 4π r² = ρ (4/3 π r³) /ε₀

E = ρ r / 3ε₀

the density is

ρ = Q / V

V = 4/3 π a³

E = Q 3 / (4π a³) r / 3ε₀

k = 1 / 4π ε₀

E = k Q r / a³

let's calculate

for r = 4.00cm = 0.04m

E = 8.99 10⁹ 3.00 10⁻⁹ 0.04 / 0.05³

E = 8.63 10³ N / c

for r = 6.00 cm

in this case the gaussine surface is outside the sphere, so all the charge is inside

E (4π r²) = Q /ε₀

E = k q / r²

let's calculate

E = 8.99 10⁹ 3 10⁻⁹ / 0.06²

E = 7.49 10³ N/C

b) We repeat in calculation for a conducting sphere.

For r = 4 cm

In this case, all the charge eta on the surface of the sphere, due to the mutual repulsion between the mobile charges, so since there is no charge inside the Gaussian surface, therefore the field is zero.

E = 0

In the case of r = 0.06 m, in this case, all the load is inside the Gaussian surface, therefore the field is

E = k q / r²

E = 7.49 10³ N / C