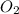Answer: 15.62 moles of
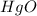 are needed to produce 250.0 g of
are needed to produce 250.0 g of
Step-by-step explanation:
To calculate the moles :
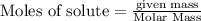
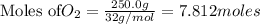
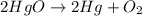
According to stoichiometry :
1 mole of
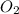 is produced from decomposition of = 2 moles of
is produced from decomposition of = 2 moles of
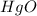
Thus 7.812 moles of
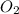 will be produced from decomposition of =
will be produced from decomposition of =
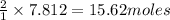 of
of
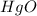
Thus 15.62 moles of
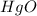 are needed to produce 250.0 g of
are needed to produce 250.0 g of