Question

A wheel rotating with a constant angular acceleration turns through 22 revolutions during a 5 s time interval. Its angular velocity at the end of this interval is 12 rad/s. What is the angular acceleration of the wheel? Note that the initial angular velocity is not zero. Answer in units of rad/s 2 .

Answer 1

0.52rad/s^2

Step-by-step explanation:

To find the angular acceleration you use the following formula:

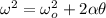`\omega^2=\omega_o^2+2\alpha\theta` (1)

w: final angular velocity

wo: initial angular velocity

θ: revolutions

α: angular acceleration

you replace the values of the parameters in (1) and calculate α:

`\alpha=(\omega^2-\omega_o^2)/(2\theta)`

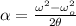
you use that θ=22 rev = 22(2π) = 44π

`\alpha=((12rad/s)^2-(0rad/s)^2)/(2(44\pi))=0.52(rad)/(s^2)`

hence, the angular acceñeration is 0.52rad/s^2