Question

A 100g sample of Carbon-14 has a half-life of 5 years. How much Carbon-14 is left after 10 years?

Answer 1

Answer: The carbon-14 left after 10 years is 25 g

Step-by-step explanation:

Expression for rate law for first order kinetics is given by:

`t=(2.303)/(k)\log(a)/(a-x)`

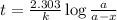
where,

k = rate constant

t = age of sample

a = let initial amount of the reactant

a - x = amount left after decay process

a) for rate constant

`t_{(1)/(2)}=(0.693)/(k)`

`k=(0.693)/(5years)=0.139years^(-1)`

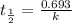
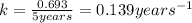
b) for amount left after 10 years

`t=(2.303)/(k)\log(a)/((a-x))`

`10=(2.303)/(0.139)\log(100)/((a-x))`

`(a-x)=25g`

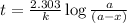
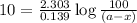
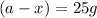
Thus carbon-14 left after 10 years is 25 g