Question

Jackie makes an $800 deposit into a bank account earning 4.5% interest.

a. If the interest is compounded quarterly how much money will Jackie have in 10 years?
b. If the interest is compounded continuously how much money will Jackie have in 10 years?
C. If Jackie's money is compounded continuously, how long will it take her money to double?
d. If Jackie's money is compounded monthly, how long will it take her money to triple?

Answer 1

a. $1251.5

b. $1254.64

c. 15.4 years

d. 30.56 years

Explanation:

Given the information:

• Principle (P) = $800
• Interest = 4.5%

As we know, the formula to find the future value of Jackie deposit with interest is compounded monthly, quaterly, ..is

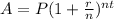`A=P(1+(r)/(n) )^(nt)` where:

• P = initial balance
• r = interest rate (decimal)
• n = number of times compounded annually
• t = time

Hence:

a. If the interest is compounded quarterly how much money will Jackie have in 10 years?

`A=P(1+(r)/(n) )^(nt)`

<=>
`A=800(1+(0.045)/(4) )^(4*10)`

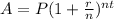
<=> A = $1251.5

d. If Jackie's money is compounded monthly, how long will it take her money to triple?

<=>
`A=P(1+(r)/(n) )^(nt) = 3*800`

<=>
`800(1+(0.045)/(12) )^(12*t) = 2400`

<=>
`(1+(0.045)/(12) )^(12*t) = 3`

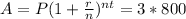
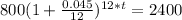
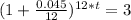
<=> t = 30.56 years

As we know, the formula to find the future value of Jackie deposit with interest is compounded continuously

A = P*
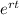`e^(rt)` where e is the mathematical constant approximated as 2.7183.

b. If the interest is compounded continuously how much money will Jackie have in 10 years?

<=> A = 800*
`2.7183^(0.045*10)`

<=> A = $1254.64

C. If Jackie's money is compounded continuously, how long will it take her money to double?

<=> P*
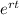`e^(rt)` = 2*800

<=> 800*
`2.7183^(0.045*t)` = 1600

<=>
`2.7183^(0.045*t)` = 2

<=> t = 15.4 years