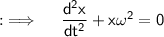Given that the position x of a particle along X-axis varies with time t by the equation:
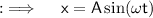
As it defines the position, so x is just displacement here, and we need to find the acceleration first for telling with what if varies, so by definition, the second differential coefficient of displacement is acceleration, so differentiating both sides w.r.t.x of the above equation in accordance with chain rule we have:
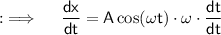
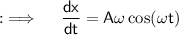
Differentiating both sides w.r.t.x by chain rule again to get the 2nd order derivative
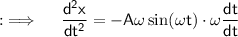

Re-write as :
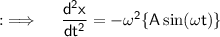
Can be further written as
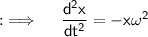
This is the Required answer
If they ask you the differential equation for the acceleration of a wave (as the given equation was general equation of a wave), you can simply write: