Question

Waitresses all want to work the "high roller" room at a local restaurant. There are six waitresses each evening assigned to one of six rooms in the restaurant. Tne room assignments are made by fair random selection such that each waitress has an equal probability of being in the "high roller" room. If a waitress in the "high roller" room will always make $200 in tips over the course of an evening while waitresses in all other rooms will make $75, over time (on average) how much can a waitress at this restaurant expect to make per evening?

Answer 1

The expected amount earned by a waitress per evening is $95.83.

Explanation:

At a local restaurant there are six rooms total; 1 is a "high roller" room and other 5 are normal rooms.

There are 6 waitresses working at the local restaurant.

The room assignments are made by fair random selection such that each waitress has an equal probability of being in the "high roller" room.

This implies that a waitress can be assigned any of the six rooms with probability,
`(1)/(6)`.

The tip earned by the waitress working in the "high roller" room is $200 and that for other rooms is $75.

So, the distribution of tips earned is as follows:

X: $200 $75 $75 $75 $75 $75

P (X):
`(1)/(6)`
`(1)/(6)`
`(1)/(6)`
`(1)/(6)`
`(1)/(6)`
`(1)/(6)`

The expected value of a random variable is given by:

`E(X)=\sum x\cdot P (X)`

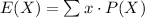
Compute the expected amount earned by a waitress per evening as follows:

`E(X)=\sum x\cdot P (X)`

`=(200* (1)/(6))+(75* (5)/(6))\\\\=(575)/(6)\\\\=95.8333\\\\\approx 95.83`

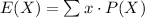
Thus, the expected amount earned by a waitress per evening is $95.83.