Question

A single-turn circular loop of wire of radius 45 mm lies in a plane perpendicular to a spatially uniform magnetic field. During a 0.10 s time interval, the magnitude of the field increases uniformly from 250 to 350 mT. (a) Determine the emf induced in the loop (in V). (Enter the magnitude.) V (b) If the magnetic field is directed out of the page, what is the direction of the current induced in the loop

Answer 1

Magnitude of induced emf is 0.00635 V

Step-by-step explanation:

Radius of circular loop r = 45 mm = 0.045 m

Area of circular loop
`A=\pi r^2`

`A=3.14* 0.045^2=0.00635m^2`

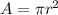
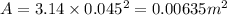
Magnetic field is increases from 250 mT to 350 mT

Therefore change in magnetic field
`dB=250-350=100mT`

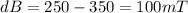
Emf induced is given by

`e=-N(d\Phi )/(dt)=-NA(dB)/(dt)`

`e=-0.00635* (100* 10^(-3))/(0.10)=-0.00635V`

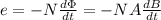
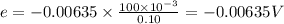
Magnitude of induced emf is equal to 0.00635 V