Question

A potential candidate for President has stated that she will run for office if at least 30% of Americans voice support for her candidacy. To make her decision she draws a random sample of 500 Americans. Suppose that in fact 35% of all Americans support her candidacy. What is the probability that the potential candidate will obtain a p^ ≥ 0.30 (and run for President)? Round your answer to four decimal places.

Answer 1

Probability that the potential candidate will run for President election is 0.0096.

Explanation:

We are given that a potential candidate for President has stated that she will run for office if at least 30% of Americans voice support for her candidacy.

To make her decision she draws a random sample of 500 Americans. Suppose that in fact 35% of all Americans support her candidacy.

Let p = % of Americans voice support for her candidacy

The z score probability distribution for sample proportion is given by;

Z =
`\frac{\hat p-p}{\sqrt{(\hat p(1-\hat p))/(n)} }` ~ N(0,1)

where,
`\hat p` = sample proportion of Americans support her candidacy = 35%

n = sample of Americans = 500

Now, probability that the potential candidate will obtain a p^ ≥ 0.30 and run for President is given by = P(
`\hat p` ≥ 0.30)

P(
`\hat p` ≥ 0.30) = P(
`\frac{\hat p-p}{\sqrt{(\hat p(1-\hat p))/(n)} }` ≥
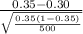`\frac{0.35-0.30}{\sqrt{(0.35(1-0.35))/(500)} }` ) = P(Z ≥ 2.34) = 1 - P(Z
`\leq` 2.34)

= 1 - 0.9904 = 0.0096

The above probability is calculated by looking at the value of x = 2.34 in the z table which has an area of 0.9904.

Hence, the required probability is 0.0096.