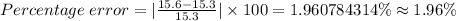Answer:
Explanation:
The error percentage is a measure of how inaccurate a measurement is, standardized based on the size of the measurement. It can be easily calculated using the following formula:
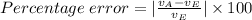
Where:

Therefore, according to the data provided by the problem:
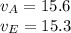
The percentage error is: