Question

An insurance company is interested in conducting a study to to estimate the population proportion of teenagers who obtain a driving permit within 1 year of their 16th birthday. A level of confidence of 99% will be used and an error of no more than .04 is desired. There is no knowledge as to what the population proportion will be. The size of sample should be at least _______. 289

Answer 1

The size of the sample should be at least 1037.

Explanation:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

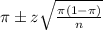
In which

z is the zscore that has a pvalue of
`1 - (\alpha)/(2)`.

The margin of error of the interval is given by:

`M = z\sqrt{(\pi(1-\pi))/(n)}`

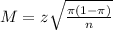
99% confidence level

So
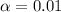`\alpha = 0.01`, z is the value of Z that has a pvalue of
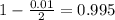`1 - (0.01)/(2) = 0.995`, so
`Z = 2.575`.

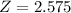A level of confidence of 99% will be used and an error of no more than .04 is desired. There is no knowledge as to what the population proportion will be. The size of sample should be at least

We need a sample size of at least n.

n is found when
`M = 0.04`

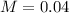
We don't know the proportion of the population, so we use
`\pi = 0.5`

`M = z\sqrt{(\pi(1-\pi))/(n)}`

`0.04 = 2.575\sqrt{(0.5*0.5)/(n)}`

`0.04√(n) = 2.575*0.5`

`√(n) = (2.575*0.5)/(0.04)`

`(√(n))^(2) = ((2.575*0.5)/(0.04))^(2)`

`n = 1036.03`

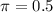
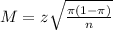
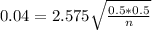
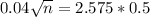
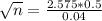
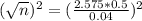
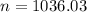
Rounding up

The size of the sample should be at least 1037.