We have been given 4 choices. We are asked to choose the volume that could belong to a cube with a side length that is an integer.
We know that volume of a cube is cube of each side length.
To solve our given problem, we will take cube root of each given value. The cube root of which value will be an integer that will be our correct choice.
A.
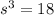
![\sqrt[3]{s^3}=\sqrt[3]{18}](https://img.qammunity.org/2021/formulas/mathematics/high-school/q7bucvutuc7rjopts3qv1r78c8ven9ibrx.png)
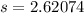
Since cube root of 18 is not an integer, therefore, 18 is not a correct choice.
B.
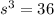
![\sqrt[3]{s^3}=\sqrt[3]{36}](https://img.qammunity.org/2021/formulas/mathematics/high-school/yd2koz16ba03ixxao11kbk85rgc8qu2cj1.png)

Since cube root of 36 is not an integer, therefore, 36 is not a correct choice.
C.

![\sqrt[3]{s^3}=\sqrt[3]{64}](https://img.qammunity.org/2021/formulas/mathematics/high-school/wk2vdfyq0p318gsa67evxx43vi2i2xv85m.png)
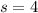
Since cube root of 64 is 4 and 4 is an integer, therefore, 64 is the correct choice.
D.
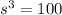
![\sqrt[3]{s^3}=\sqrt[3]{100}](https://img.qammunity.org/2021/formulas/mathematics/high-school/h6do5yh8couo7yq52kal1u9fp56pa3b786.png)
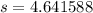
Since cube root of 100 is not an integer, therefore, 100 is not a correct choice.