Question

Gina and Stewart are surf-fishing on the Atlantic coast, where both bluefish and pompano are common catches. The mean length of a bluefish is 264 millimeters with a standard deviation of 57mm. For pompano, the mean is 157mm with a standard deviation of 28mm.

Stewart caught a bluefish that was 283mm long, and Gina caught a pompano that was 152mm long. Who caught the longer fish, relative to fish of the same species?

Answer 1

Due to the higher z-score, Stewart caught the longer fish, relative to fish of the same species

Explanation:

Problems of normally distributed samples are solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

Who caught the longer fish, relative to fish of the same species?

Whosoever fish's had the higher z-score.

Stewart caught a bluefish that was 283mm

The mean length of a bluefish is 264 millimeters with a standard deviation of 57mm.

So we have to find Z when
`X = 283, \mu = 264, \sigma = 57`

`Z = (X - \mu)/(\sigma)`

`Z = (283 - 264)/(57)`

`Z = 0.33`

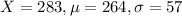
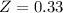
Gina caught a pompano that was 152mm long.

For pompano, the mean is 157mm with a standard deviation of 28mm.

So we have to find Z when
`X = 152, \mu = 157, \sigma = 28`

`Z = (X - \mu)/(\sigma)`

`Z = (152 - 157)/(28)`

`Z = -0.18`

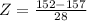
Due to the higher z-score, Stewart caught the longer fish, relative to fish of the same species