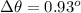Answer:
The angle of separation is
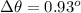
Step-by-step explanation:
From the question we are told that
The angle of incidence is
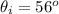
The refractive index of violet light in diamond is
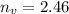
The refractive index of red light in diamond is
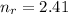
The wavelength of violet light is
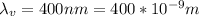
The wavelength of red light is
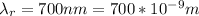
Snell's Law can be represented mathematically as
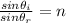
Where
 is the angle of refraction
is the angle of refraction
=>
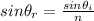
Now considering violet light
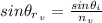
substituting values
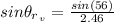

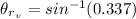
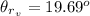
Now considering red light
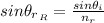
substituting values
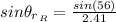

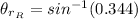

The angle of separation between the red light and the violet light is mathematically evaluated as
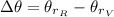
substituting values