Question

An airplane is traveling at a fixed altitude with an outside wind factor. The airplane is headed N 40° W at a speed of 600 miles per hour. As the airplane comes to a certain point, it comes across a wind in the direction N 45° E with a velocity of 80 miles per hour. What are the resultant speed and direction of the airplane? Round your answers to the nearest hundredth.

Answer 1

Answer:
`\vec{v_R}=\hat{i}[-329.11]+\hat{j}[516.18]`

Step-by-step explanation:

Given

Plane is initially flying with velocity of magnitude
`v=600\ mph`

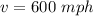
at angle of
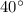`40^(\circ)` with North towards west

Velocity of plane airplane can be written as

`v_a=600(-\sin 40\hat{i}+\cos 40\hat{j})`

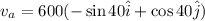
Now wind is encountered with speed of
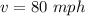`v=80\ mph` at angle of
`N45^(\circ)E`

`v_w=80(\cos 45\hat{i}+\sin 45\hat{j})`

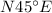
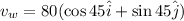
resultant velocity

`\vec{v_R}=\vec{v_a} +\vec{v_w}`

`\vec{v_R}=600(-\sin 40\hat{i}+\cos 40\hat{j})+ 80(\cos 45\hat{i}+\sin 45\hat{j})`

`\vec{v_R}=\hat{i}[-385.67+56.56]+\hat{j}[459.62+56.56]`

`\vec{v_R}=\hat{i}[-329.11]+\hat{j}[516.18]`

for direction
`\tan \theta =(516.18)/(329.11)`

`\tan \theta =1.568`

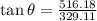
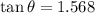
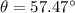`\theta =57.47^(\circ)` west of North