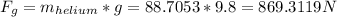Question

A helium balloon ride lifts up passengers in a basket. Assume the density of air is 1.28 kg/m3 and the density of helium in the balloon is 0.18 kg/m3. The radius of the balloon (when filled) is R = 4.9 m. The total mass of the empty balloon and basket is mb = 121 kg and the total volume is Vb = 0.073 m3. Assume the average person that gets into the balloon has a mass mp = 73 kg and volume Vp = 0.077 m3. 1)What is the volume of helium in the balloon when fully inflated? m3 2)What is the magnitude of the force of gravity on the entire system (but with no people)? Include the mass of the balloon, basket, and helium. N

Answer 1

1) The volume of helium in the ballon when is fully inflated is 492.8070 m³

2) The magnitude of the force of gravity (with no people) is 869.3119 N

Step-by-step explanation:

Given:

ρair = density of air = 1.28 kg/m³

ρhelium = density of helium = 0.18 kg/m³

R = radius of balloon = 4.9 m

mtotal = 121 kg

Vtotal = 0.073 m³

mp = average mass per person = 73 kg

Vp = 0.077 m³

g = gravity = 9.8 m/s²

Questions:

1) What is the volume of helium in the balloon when fully inflated, Vhelium = ?

2) What is the magnitude of the force of gravity on the entire system (but with no people), Fg = ?

1) The volume of helium in the ballon when is fully inflated

`V_(helium) =(4)/(3) \pi R^(3) =(4)/(3) \pi *4.9^(3) =492.8070m^(3)`

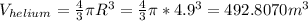
2) First, you need to calculate the mass of helium

`m_(helium) =\rho _(helium) *V_(helium) =0.18*492.8070=88.7053kg`

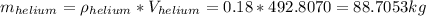
The magnitude of the force of gravity (with no people)

`F_(g) =m_(helium) *g=88.7053*9.8=869.3119N`