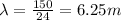Answer:
Mass of the rope m = 6.272 kg
Wavelength is equal to 6.25 m
Step-by-step explanation:
Length of pulley L = 180 M
Mass at the end of pulley m = 180 kg
Frequency is given f = 24 Hz
Acceleration due to gravity
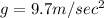
Tension
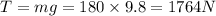
Velocity of wave in the rope v = 150 m/sec
Velocity of wave is given by
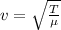
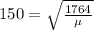
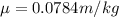
Therefore mass of the rope
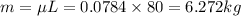
Wavelength is equal to