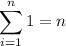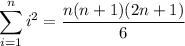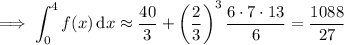Split up the interval [0, 4] into 6 subintervals of equal length (4-0)/6 = 2/3:
[0, 2/3], [2/3, 4/3], [4/3, 2], [2, 8/3], [8/3, 10/3], [10/3, 4]
The right endpoints form an arithmetic sequence,
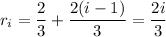
where
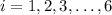 .
.
We estimate the area under the curve over each subinterval by a rectangle with length 2/3 and height equal to the value of the function at the right endpoint. Then the value of the integral is approximately equal to the sum of these rectangles' areas:

We have
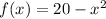 , so
, so

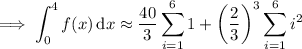
Recall that