Answer:
Proof in explanation.
Explanation:
I'm going to assume you mean "greater than or equal to".
We know
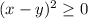 .
.
We know this because any real number squared will result in a positive or zero result.
Let's expand the left hand side:
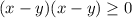
Distribute:
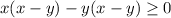
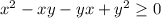
Combine like terms:
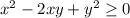
Commutative property of addition:
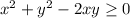
Add
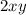 on both sides:
on both sides:
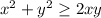
Now we wanted to show
 . Our right hand side almost appeared that way except the factor of 2 present there.
. Our right hand side almost appeared that way except the factor of 2 present there.
Let's go back to our inequality that we got:
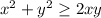
Divide both sides by 2:
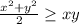
Note: Half of a positive number is less than that positive number.

This inequality says we would like it to say in the end:
