Question

Listed below are the lead concentrations in mu ??g/g measured in different traditional medicines. Use a 0.10 0.10 significance level to test the claim that the mean lead concentration for all such medicines is less than 17 17 mu ??g/g. 6.5 18.5?21.5?5.5?8.5?4.5?4.5?17.5 15.5 20

What are the null and alternative? hypotheses?

A. Upper H0?: mu ? =17 mu ??g/g Upper H1?: mu ? ? 17 mu ??g/g

B. Upper H0?: mu > 17 mu ??g/g Upper H1?: mu ? < 17 mu ??g/g

C. Upper H0?: mu ? = 17 mu ??g/g Upper H1?: mu ? < 17 mu ??g/g

D. Upper H0?: mu ? = 17 mu ??g/g Upper H1?: mu ? > 17 mu ??g/g

Determine the test statistic. nothing ?(Round to two decimal places as? needed.)

Determine the? P-value. nothing ?(Round to three decimal places as? needed.)

State the final conclusion that addresses the original claim. ? Fail to reject Reject Upper H 0 H0. There is ? sufficient not sufficient evidence to conclude that the mean lead concentration for all such medicines is ? less than equal to greater than not 17 17 mu ??g/g.

Answer 1

We conclude that the mean lead concentration for all such medicines is less than 17 mu.

Explanation:

We are given below are the lead concentrations in mu;

6.5 18.5 21.5 5.5 8.5 4.5 4.5 17.5 15.5 20

We have to test the claim that the mean lead concentration for all such medicines is less than 17 mu.

Let
`\mu` = mean lead concentration for all such medicines.

So, Null Hypothesis,
`H_0` :
`\mu` = 17 mu {means that mean lead concentration for all such medicines is equal to 17 mu}

Alternate Hypothesis,
`H_A` :
`\mu` < 17 mu {means that the mean lead concentration for all such medicines is less than 17 mu}

The test statistics that would be used here One-sample t test statistics as we don't know about population standard deviation;

T.S. =
`(\bar X-\mu)/((s)/(√(n)))` ~
`t_n_-_1`

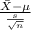where,
`\bar X` = sample mean lead concentration =
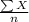`(\sum X)/(n)` = 12.25 mu

s = sample standard deviation =
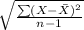`\sqrt{(\sum (X-\bar X)^(2) )/(n-1) }` = 6.96 mu

n = sample size = 10

So, test statistics =
`(12.25 -17)/((6.96)/(√(10)))` ~
`t_9`

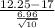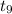
= -2.16

The value of t test statistics is -2.16.

Now, the P-value of the test statistics is given by the following formula;

P-value = P(
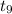`t_9` < -2.16) = 0.031

Now, at 0.10 significance level the t table gives critical value of -1.383 for left-tailed test. Since our test statistics is less than the critical value of t as -2.16 < -1.383, so we have sufficient evidence to reject our null hypothesis as it will fall in the rejection region due to which we reject our null hypothesis.

Therefore, we conclude that the mean lead concentration for all such medicines is less than 17 mu.