Question

If Jessica is challenged to a game of Sums, which statement below is accurate in every aspect in guiding her to the correct choice of choosing to play odds or evens? E(evens) will be more because there are more even numbers that result from rolling two dice. Therefore, Jessica should play evens. E(odds) will be more because the probability for each odd number being rolled is greater. Therefore, Jessica should play odds. E(evens) will be more because the value of the even numbers on the dice are more. Therefore, Jessica should play evens. E(evens) = E(odds) because the different probabilities and values end up balancing out, creating a fair game. Therefore, Jessica may choose whichever she likes.

Answer 1

Answer: the answer would be d

Step-by-step explanation: correct answer on edg!

Answer 2

E(evens) = E(odds) because the different probabilities and values end up balancing out, creating a fair game. Therefore, Jessica may choose whichever she likes.

Explanation:

Jessica is challenged to a game of sums.

Which means,

E(Dice 1) + E(Dice 2)

Let's first check the sum of all possible numbers of the two dice.

We have:

(1+1 = 2); (1+2=3); (1+3=4); (1+4+5); (1+5=6); (1+6=7); (2+1=3); (2+2=4); (2+3=5); (2+4=6); (2+5=7); (2+6=8); (3+1=4); (3+2=5); (3+3=6); (3+4=7); (3+5=8); (3+6=9); (4+1=5); (4+2=6); (4+3=7); (4+4=8); (4+5=9); (4+6=10); (5+1=6); (6+1=7); (6+2=8);*(6+3=9); (6+4=10); (6+5=11); (6+6+12);

Total possible outcome = 36 results.

(2, 3, 4, 5, 6, 7, 3, 4, 5, 6, 7, 8, 4, 5, 6, 7, 8, 9, 5, 6, 7, 8, 9, 10, 6, 7, 8, 9, 10, 11, 7, 8, 9, 10, 11, 12)

Probability of even numbers =

E(even) =
`(18)/(36)`

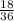
Probability of odd numbers =

E(odd) =
`(18)/(36)`

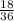
Since they are equal,

E(even) = E(odd)

The correct choice for Jessica is:

E(evens) = E(odds) because the different probabilities and values end up balancing out, creating a fair game. Therefore, Jessica may choose whichever she likes.