Question

A manufacturer of banana chips would like to know whether its bag filling machine works correctly at the 402 gram setting. It is believed that the machine is underfilling the bags. A 25 bag sample had a mean of 393 grams with a standard deviation of 20. Assume the population is normally distributed. A level of significance of 0.02 will be used. Find the value of the test statistic. Round your answer to three decimal places.

Answer 1

The value of t test statistics is -2.25.

Explanation:

We are given that a manufacturer of banana chips would like to know whether its bag filling machine works correctly at the 402 gram setting. It is believed that the machine is under filling the bags.

A 25 bag sample had a mean of 393 grams with a standard deviation of 20.

Let
`\mu` = mean filling of bags by the machine.

So, Null Hypothesis,
`H_0` :
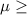`\mu \geq` 402 gram {means that the the machine is not under filling the bags}

Alternate Hypothesis,
`H_A` :
`\mu` < 402 gram {means that the the machine is under filling the bags}

The test statistics that would be used here One-sample t test statistics as we don't know about the population standard deviation;

T.S. =
`(\bar X-\mu)/((s)/(√(n) ) )` ~
`t_n_-_1`

where,
`\bar X` = sample mean bag filling = 393 grams

s = sample standard deviation = 20 grams

n = sample of bags = 25

So, test statistics =
`(393-402)/((20)/(√(25) ) )` ~
`t_2_4`

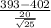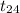
= -2.25

The value of t test statistics is -2.25.