Answer:
(a) The probability that the sample average will be between $30.00 and $31.00 is 0.5539.
(b) The probability that the sample average will exceed $21.00 is 0.12924.
(c) The probability that the sample average will be less than $22.80 is 0.04006.
Explanation:
We are given that the hourly wage was $30.67 for Switzerland, $20.20 for Japan, and $23.82 for the U.S.
Assume that in all three countries, the standard deviation of hourly labor rates is $4.00.
(a) Suppose 40 manufacturing workers are selected randomly from across Switzerland.
Let
 = sample average wage
= sample average wage
The z score probability distribution for sample mean is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean wage for Switzerland = $30.67
= population mean wage for Switzerland = $30.67
 = standard deviation = $4.00
= standard deviation = $4.00
n = sample of workers selected from across Switzerland = 40
Now, the probability that the sample average will be between $30.00 and $31.00 is given by = P($30.00 <
 < $31.00)
< $31.00)
P($30.00 <
 < $31.00) = P(
< $31.00) = P(
 < $31.00) - P(
< $31.00) - P(

 $30.00)
$30.00)
P(
 < $31) = P(
< $31) = P(
 <
<
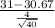 ) = P(Z < 0.52) = 0.69847
) = P(Z < 0.52) = 0.69847
P(

 $30) = P(
$30) = P(


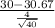 ) = P(Z
) = P(Z
 -1.06) = 1 - P(Z < 1.06)
-1.06) = 1 - P(Z < 1.06)
= 1 - 0.85543 = 0.14457
The above probability is calculated by looking at the value of x = 0.52 and x = 1.06 in the z table which has an area of 0.69847 and 0.85543 respectively.
Therefore, P($30.00 <
 < $31.00) = 0.69847 - 0.14457 = 0.5539
< $31.00) = 0.69847 - 0.14457 = 0.5539
(b) Suppose 32 manufacturing workers are selected randomly from across Japan.
Let
 = sample average wage
= sample average wage
The z score probability distribution for sample mean is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean wage for Japan = $20.20
= population mean wage for Japan = $20.20
 = standard deviation = $4.00
= standard deviation = $4.00
n = sample of workers selected from across Japan = 32
Now, the probability that the sample average will exceed $21.00 is given by = P(
 > $21.00)
> $21.00)
P(
 > $21) = P(
> $21) = P(
 >
>
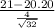 ) = P(Z > 1.13) = 1 - P(Z < 1.13)
) = P(Z > 1.13) = 1 - P(Z < 1.13)
= 1 - 0.87076 = 0.12924
The above probability is calculated by looking at the value of x = 1.13 in the z table which has an area of 0.87076.
(c) Suppose 47 manufacturing workers are selected randomly from across United States.
Let
 = sample average wage
= sample average wage
The z score probability distribution for sample mean is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean wage for United States = $23.82
= population mean wage for United States = $23.82
 = standard deviation = $4.00
= standard deviation = $4.00
n = sample of workers selected from across United States = 47
Now, the probability that the sample average will be less than $22.80 is given by = P(
 < $22.80)
< $22.80)
P(
 < $22.80) = P(
< $22.80) = P(
 <
<
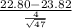 ) = P(Z < -1.75) = 1 - P(Z
) = P(Z < -1.75) = 1 - P(Z
 1.75)
1.75)
= 1 - 0.95994 = 0.04006
The above probability is calculated by looking at the value of x = 1.75 in the z table which has an area of 0.95994.