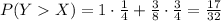Question

Let X equal the number of hours that a randomly selected person from City A reads the news online each day. Suppose that X has a continuous uniform distribution on the interval [1, 4]. Let Y equal the number of hours that a randomly selected person from City B reads the news online each day. Suppose that Y has a continuous uniform distribution on the interval [1,5]. Assume that X and Y are independent. Determine P(Y> X).

Answer 1

`P(Y>X) = (17)/(32)`

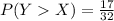
Explanation:

Recall that since X is uniformly distributed over the set [1,4] we have that the pdf of X is given by
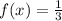`f(x) = (1)/(3)` if
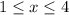`1\leq x \leq 4` and 0 otherwise. In the same manner, the pdf of Y is given by
`g(y) = (1)/(4)` if
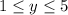`1\leq y\leq 5` and 0 otherwise.

Note that if Y is in the interval (4,5] then Y>X by default. So, in this case we have that P(Y>X| y in (4,5]) = 1. We want to calculate the probability of having Y in that interval . That is

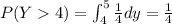`P(Y>4) = \int_(4)^(5)(1)/(4)dy = (1)/(4)`. Thus,
`P(Y\leq 4 ) = 1 - P(Y>4)= (3)/(4)`.

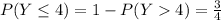We want to proceed as follows. Using the total probability theorem, given two events A, B we have that

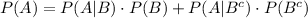`P(A) = P(A|B)\cdot P(B) + P(A|B^c) \cdot P(B^c)` In this case, A is the event that Y>X and B is the event that Y is in the interval (4,5].

If we assume that X and Y are independent, then we have that the joint pdf of X,Y is given by
`h(x,y) = f(x)g(y) = (1)/(12)` when
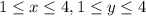`1\leq x \leq 4, 1\leq y \leq 4`. We can draw the region were Y>X and the function h(x,y) is different from 0. (The drawing is attached). This region is described as follows:
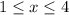`1\leq x \leq 4` and
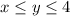`x\leq y \leq 4`, then (the specifics of the calculations of the integrals are ommitted)

`P(Y>X| y \in [1,4)) = \int_(1)^(4)\int_(x)^(4)(1)/(12)dy dx = (1)/(12)\int_(1)^(4) (4-x) dx = (1)/(12)\left.(4x-(x)/(2))\right|_(1)^(4)= (1)/(12)(4\cdot 4 - (4^2)/(2)-(4-(1)/(2)) = (9)/(2\cdot 12) = (3)/(8)`

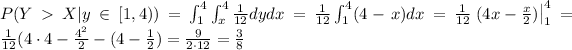
Thus,

`P(Y>X) = 1\cdot (1)/(4) + (3)/(8)\cdot (3)/(4) = (17)/(32)`