Question

Assume that you have a sample of n 1 equals 6​, with the sample mean Upper X overbar 1 equals 50​, and a sample standard deviation of Upper S 1 equals 7​, and you have an independent sample of n 2 equals 5 from another population with a sample mean of Upper X overbar 2 equals 38 and the sample standard deviation Upper S 2 equals 8. Assuming the population variances are​ equal, at the 0.01 level of​ significance, is there evidence that mu 1 greater than mu 2​?

Answer 1

`t=\frac{(50 -38)-(0)}{7.46\sqrt{(1)/(6)+(1)/(5)}}=2.656`

`df=6+5-2=9`

`p_v =P(t_(9)>2.656) =0.0131`

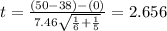
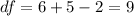
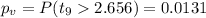
Since the p value is higher than the significance level given of 0.01 we don't have enough evidence to conclude that the true mean for group 1 is significantly higher thn the true mean for the group 2.

Explanation:

Data given

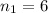`n_1 =6` represent the sample size for group 1

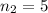`n_2 =5` represent the sample size for group 2

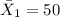`\bar X_1 =50` represent the sample mean for the group 1

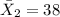`\bar X_2 =38` represent the sample mean for the group 2

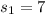`s_1=7` represent the sample standard deviation for group 1

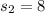`s_2=8` represent the sample standard deviation for group 2

System of hypothesis

The system of hypothesis on this case are:

Null hypothesis:
`\mu_1 \leq \mu_2`

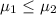
Alternative hypothesis:
`\mu_1 > \mu_2`

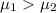
We are assuming that the population variances for each group are the same

`\sigma^2_1 =\sigma^2_2 =\sigma^2`

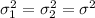
The statistic for this case is given by:

`t=\frac{(\bar X_1 -\bar X_2)-(\mu_(1)-\mu_2)}{S_p\sqrt{(1)/(n_1)+(1)/(n_2)}}`

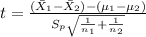
The pooled variance is:

`S^2_p =((n_1-1)S^2_1 +(n_2 -1)S^2_2)/(n_1 +n_2 -2)`

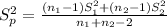
We can find the pooled variance:

`S^2_p =((6-1)(7)^2 +(5 -1)(8)^2)/(6 +5 -2)=55.67`

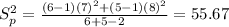
And the pooled deviation is:

`S_p=7.46`

The statistic is given by:

`t=\frac{(50 -38)-(0)}{7.46\sqrt{(1)/(6)+(1)/(5)}}=2.656`

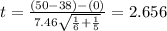
The degrees of freedom are given by:

`df=6+5-2=9`

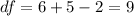
The p value is given by:

`p_v =P(t_(9)>2.656) =0.0131`

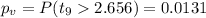
Since the p value is higher than the significance level given of 0.01 we don't have enough evidence to conclude that the true mean for group 1 is significantly higher thn the true mean for the group 2.