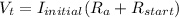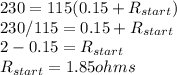Answer:
a)
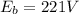
b) P = 13,800 W
c)

di)
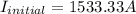
dii)
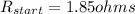
Step-by-step explanation:
Voltage, Vt = 230 V
Armature current,

Armature Resistance,
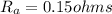
a) The back emf is calculated as follows:
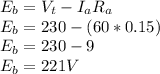
b) The power supplied to the armature (W)

P = 230 * 60
P = 13,800 W
c) Mechanical power developed by the motor
 = Power supplied to the armature - Power lost in the armature
= Power supplied to the armature - Power lost in the armature
Power lost in the armature,
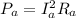
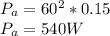
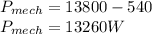
d)( i)The initial starting current if the motor is directly connected across the 230 V line
At starting, there is no back emf,
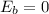


ii) Value of the starting resistor needed to limit the initial current to 115 A